imported>Paul Wormer |
imported>Paul Wormer |
| (56 intermediate revisions by the same user not shown) |
| Line 1: |
Line 1: |
| ==Rotations in <math>\mathbb{R}^3</math> == | | ==Parabolic mirror== |
| Consider a real 3×3 matrix '''R''' with columns
| | {{Image|Refl parab.png|right|350px|Fig. 2. Reflection in a parabolic mirror}} |
| '''r'''<sub>1</sub>, '''r'''<sub>2</sub>, '''r'''<sub>3</sub>,
| | Parabolic mirrors concentrate incoming vertical light beams in their focus. We show this. |
| i.e.,
| |
| :<math>
| |
| \mathbf{R} = \left(\mathbf{r}_1 , \mathbf{r}_2 , \mathbf{r}_3 \right)
| |
| </math>.
| |
| The matrix '''R''' is ''orthogonal'' if
| |
| :<math>
| |
| \mathbf{r}_i \cdot \mathbf{r}_j = \delta_{ij}, \quad i,j = 1,2,3 .
| |
| </math>
| |
| The matrix '''R''' is a ''proper rotation matrix'', if it is
| |
| orthogonal ''and'' if '''r'''<sub>1</sub>, '''r'''<sub>2</sub>,
| |
| '''r'''<sub>3</sub> form a right-handed set, i.e.,
| |
| :<math>
| |
| \mathbf{r}_i \times \mathbf{r}_j = \sum_{k=1}^3 \, \varepsilon_{ijk}
| |
| \mathbf{r}_k .
| |
| </math>
| |
| Here the symbol × indicates a
| |
| [[cross product]] and <math>\varepsilon_{ijk}</math> is the
| |
| antisymmetric [[Levi-Civita symbol]],
| |
| :<math>
| |
| \begin{align}
| |
| \varepsilon_{123} =&\; \varepsilon_{312} = \varepsilon_{231} = 1 \\
| |
| \varepsilon_{213} =&\; \varepsilon_{321} = \varepsilon_{132} = -1
| |
| \end{align}
| |
| </math>
| |
| and <math>\varepsilon_{ijk} = 0</math> if two or more indices are equal.
| |
|
| |
|
| The matrix '''R''' is an ''improper rotation matrix'' if
| | Consider in figure 2 the arbitrary vertical light beam (blue, parallel to the ''y''-axis) that enters the parabola and hits it at point ''P'' = (''x''<sub>1</sub>, ''y''<sub>1</sub>). The parabola (red) has focus in point ''F''. The incoming beam is reflected at ''P'' obeying the well-known law: incidence angle is angle of reflection. The angles involved are with the line ''APT'' which is tangent to the parabola at point ''P''. It will be shown that the reflected beam passes through ''F''. |
| its column vectors form a left-handed set, i.e.,
| |
| :<math>
| |
| \mathbf{r}_i \times \mathbf{r}_j = - \sum_k \, \varepsilon_{ijk}
| |
| \mathbf{r}_k \; .
| |
| </math> | |
| The last two equations can be condensed into one equation
| |
| :<math>
| |
| \mathbf{r}_i \times \mathbf{r}_j = \det(\mathbf{R}) \sum_{k=1}^3 \;
| |
| \varepsilon_{ijk} \mathbf{r}_k
| |
| </math> | |
| by virtue of the the fact that
| |
| the determinant of a proper rotation matrix is 1 and of an improper
| |
| rotation −1. This can be proved as follows:
| |
| The determinant of a 3×3 matrix with column vectors '''a''', | |
| '''b''', and '''c''' can be written as | |
| :<math>
| |
| \mathbf{a} \cdot (\mathbf{b}\times\mathbf{c})
| |
| </math>.
| |
| Remember that for a proper rotation
| |
| the columns of '''R''' are orthonormal and satisfy, | |
| :<math>
| |
| \mathbf{r}_1 \cdot (\mathbf{r}_2 \times \mathbf{r}_3 ) = \sum_k \,
| |
| \varepsilon_{23k} \,
| |
| \mathbf{r}_1 \cdot \mathbf{r}_k = \varepsilon_{231} = 1 .
| |
| </math>
| |
| Likewise the determinant is −1 for an improper rotation, which ends the
| |
| proof.
| |
|
| |
|
| ====Theorem====
| | Clearly ∠''BPT'' = ∠''QPA'' (they are vertically opposite angles). Further ∠''APQ'' = ∠''FPA'' because the triangles ''FPA'' and ''QPA'' are congruent and hence ∠''FPA'' = ∠''BPT''. |
| A proper rotation matrix '''R''' can be
| |
| factorized thus
| |
| :<math>
| |
| \mathbf{R} = \mathbf{R}_z (\omega_3 ) \; \mathbf{R}_y (\omega_2 ) \; \mathbf{R}_x (\omega_1 )
| |
| </math>
| |
| which is referred to as the ''z-y-x'' parametrization,
| |
| or also as
| |
| :<math>
| |
| \mathbf{R} = \mathbf{R}_z (\alpha) \; \mathbf{R}_y (\beta ) \; \mathbf{R}_z (\gamma ) \quad
| |
| </math>
| |
| the ''z-y-z Euler parametrization''.
| |
|
| |
|
| Here
| | We prove the congruence of the triangles: By the definition of the parabola the line segments ''FP'' and ''QP'' are of equal length, because the length of the latter segment is the distance of ''P'' to the directrix and the length of ''FP'' is the distance of ''P'' to the focus. The point ''F'' has the coordinates (0,''f'') and the point ''Q'' has the coordinates (''x''<sub>1</sub>, −''f''). The line segment ''FQ'' has the equation |
| :<math> | |
| \mathbf{R}_z (\varphi ) \equiv
| |
| \begin{pmatrix}
| |
| \cos \varphi & -\sin \varphi & 0 \\
| |
| \sin \varphi & \cos \varphi & 0 \\
| |
| 0 & 0 & 1 \\
| |
| \end{pmatrix}, \quad
| |
| \mathbf{R}_y (\varphi ) \equiv
| |
| \begin{pmatrix}
| |
| \cos \varphi & 0 & \sin \varphi \\
| |
| 0 & 1 & 0 \\
| |
| -\sin \varphi& 0 & \cos \varphi \\
| |
| \end{pmatrix}, \quad
| |
| \mathbf{R}_x (\varphi ) \equiv
| |
| \begin{pmatrix}
| |
| 1 & 0 & 0 \\
| |
| 0 & \cos \varphi & -\sin \varphi \\
| |
| 0 & \sin \varphi & \cos \varphi \\
| |
| \end{pmatrix} .
| |
| </math>
| |
| ====Proof====
| |
| First the ''z-y-x''-parametrization will be proved by describing an
| |
| algorithm for the factorization of '''R'''.
| |
| Consider to that end
| |
| :<math>
| |
| \mathbf{R}_z (\omega_3 ) \, \mathbf{R}_y (\omega_2 ) =
| |
| \begin{pmatrix}
| |
| \cos \omega_3 \cos \omega_2 & -\sin \omega_3 & \cos \omega_3 \sin \omega_2 \\
| |
| \sin \omega_3 \cos \omega_2 & \cos \omega_3 & \sin \omega_3 \sin \omega_2 \\
| |
| -\sin \omega_2 & 0 & \cos \omega_2 \\
| |
| \end{pmatrix} \equiv
| |
| (\mathbf{a}_1 , \mathbf{a}_2 , \mathbf{a}_3 ) .
| |
| </math>
| |
| Note that the multiplication by
| |
| '''R'''<sub>''x''</sub>(ω<sub>1</sub>) on the right | |
| does not affect the first column, so that '''r'''<sub>1</sub> =
| |
| '''a'''<sub>1</sub>. | |
| Solve <math>\omega_2</math> and <math>\omega_3</math> from the first column of
| |
| '''R''', | |
| :<math>
| |
| \mathbf{r}_1 =
| |
| \begin{pmatrix}
| |
| \cos \omega_3 \; \cos \omega_2 \\
| |
| \sin \omega_3 \; \cos \omega_2 \\
| |
| -\sin \omega_2 \\
| |
| \end{pmatrix}.
| |
| </math>
| |
| This is possible. First solve <math>\omega_2</math> for <math> -\pi/2 \leq \omega_2
| |
| \leq \pi/2</math> from
| |
| :<math> | | :<math> |
| \sin \omega_2 = - R_{31} \equiv - (\mathbf{r}_1 )_3. | | \lambda\begin{pmatrix}0\\ f\end{pmatrix} + (1-\lambda)\begin{pmatrix}x_1\\ -f\end{pmatrix}, \quad 0\le\lambda\le 1. |
| </math> | | </math> |
| Then solve <math>\omega_3</math> for <math>0 \leq \omega_3 \leq 2 \pi</math> from
| | The midpoint ''A'' of ''FQ'' has coordinates (λ = ½): |
| :<math> | | :<math> |
| \begin{align} | | \frac{1}{2}\begin{pmatrix}0\\ f\end{pmatrix} + \frac{1}{2}\begin{pmatrix}x_1\\ -f\end{pmatrix} = |
| \cos \omega_3 =& {R_{11} \over \cos \omega_2} \\ | | \begin{pmatrix}\frac{1}{2} x_1\\ 0\end{pmatrix}. |
| \sin \omega_3 =& {R_{21} \over \cos \omega_2} . | |
| \end{align} | |
| </math> | | </math> |
| This determines the vectors '''a'''<sub>2</sub> and
| | Hence ''A'' lies on the ''x''-axis. |
| '''a'''<sub>3</sub>. | | The parabola has equation, |
| | |
| Since '''a'''<sub>1</sub>, '''a'''<sub>2</sub> and
| |
| '''a'''<sub>3</sub> are the columns of a
| |
| proper rotation matrix they form an orthonormal
| |
| right-handed
| |
| system. The plane spanned by '''a'''<sub>2</sub> and
| |
| '''a'''<sub>3</sub> is orthogonal to
| |
| <math> \mathbf{a}_1 \equiv \mathbf{r}_1</math>
| |
| and hence contains <math>\mathbf{r}_2</math> and
| |
| <math>\mathbf{r}_3</math>. Thus,
| |
| :<math> | | :<math> |
| ( \mathbf{r}_2 , \mathbf{r}_3 ) = (\mathbf{a}_2 , \mathbf{a}_3 )
| | y = \frac{1}{4f} x^2. |
| \begin{pmatrix}
| |
| \cos \omega_1 & -\sin \omega_1 \\
| |
| \sin \omega_1 & \cos \omega_1 \\
| |
| \end{pmatrix} .
| |
| </math> | | </math> |
| Since <math>\mathbf{r}_2 , \mathbf{a}_2 , \mathbf{a}_3</math> are
| | The equation of the tangent at ''P'' is |
| known unit vectors we can compute
| |
| :<math> | | :<math> |
| \begin{align} | | y = y_1 + \frac{x_1}{2f} (x-x_1)\quad \hbox{with}\quad y_1 = \frac{x_1^2}{4f}. |
| \mathbf{a}_2 \cdot \mathbf{r}_2 =& \cos \omega_1 \\
| |
| \mathbf{a}_3 \cdot \mathbf{r}_2 =& \sin \omega_1. | |
| \end{align}
| |
| </math> | | </math> |
| These equations give <math>\omega_1</math> with <math> 0 \leq \omega_1 \leq 2 \pi</math>.
| | This line intersects the ''x''-axis at ''y'' = 0, |
| Augment the matrix to <math>\mathbf{r}_x(\omega_1)</math>, then
| |
| :<math> | | :<math> |
| \begin{align} | | 0 = \frac{x_1^2}{4f} - \frac{x_1^2}{2f} + \frac{x_1}{2f} x |
| \mathbf{R} \equiv& ( \mathbf{r}_1 , \mathbf{r}_2 , \mathbf{r}_3 ) = (
| | \Longrightarrow \frac{x_1}{2f} x = \frac{x_1^2}{4f} \longrightarrow x = \tfrac{1}{2}x_1. |
| \mathbf{r}_1 , \mathbf{a}_2 , \mathbf{a}_3 )
| |
| \mathbf{R}_x (\omega_1 ) \\
| |
| =& (\mathbf{a}_1, \mathbf{a}_2, \mathbf{a}_3)\mathbf{R}_x (\omega_1 ) | |
| = \mathbf{R}_z (\omega_3 ) \, \mathbf{R}_y (\omega_2 ) \, \mathbf{R}_x (\omega_1 ) . | |
| \end{align}
| |
| </math> | | </math> |
| This concludes the proof of the ''z-y-x'' parametrization.
| | The intersection of the tangent with the ''x''-axis is the point ''A'' = (½''x''<sub>1</sub>, 0) that lies on the midpoint of ''FQ''. The corresponding sides of the triangles ''FPA'' and ''QPA'' are of equal length and hence the triangles are congruent. |
Parabolic mirror
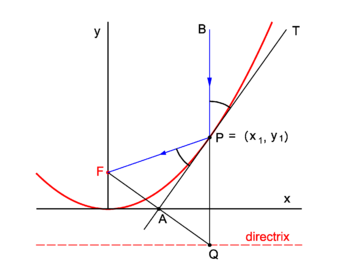
PD Image Fig. 2. Reflection in a parabolic mirror
Parabolic mirrors concentrate incoming vertical light beams in their focus. We show this.
Consider in figure 2 the arbitrary vertical light beam (blue, parallel to the y-axis) that enters the parabola and hits it at point P = (x1, y1). The parabola (red) has focus in point F. The incoming beam is reflected at P obeying the well-known law: incidence angle is angle of reflection. The angles involved are with the line APT which is tangent to the parabola at point P. It will be shown that the reflected beam passes through F.
Clearly ∠BPT = ∠QPA (they are vertically opposite angles). Further ∠APQ = ∠FPA because the triangles FPA and QPA are congruent and hence ∠FPA = ∠BPT.
We prove the congruence of the triangles: By the definition of the parabola the line segments FP and QP are of equal length, because the length of the latter segment is the distance of P to the directrix and the length of FP is the distance of P to the focus. The point F has the coordinates (0,f) and the point Q has the coordinates (x1, −f). The line segment FQ has the equation

The midpoint A of FQ has coordinates (λ = ½):

Hence A lies on the x-axis.
The parabola has equation,

The equation of the tangent at P is

This line intersects the x-axis at y = 0,

The intersection of the tangent with the x-axis is the point A = (½x1, 0) that lies on the midpoint of FQ. The corresponding sides of the triangles FPA and QPA are of equal length and hence the triangles are congruent.





