imported>Paul Wormer |
imported>Paul Wormer |
| (47 intermediate revisions by the same user not shown) |
| Line 1: |
Line 1: |
| <font style = "font-size: 85%; font-style: oblique; font-weight: bolder" >
| | ==Parabolic mirror== |
| :The Jahn-Teller effect has a quantum mechanical origin and no classical physics description of it exists. Some knowledge of quantum mechanics is prerequisite to the reading of this article. Further some chemically oriented group theory ([[Schönflies]] notation for [[point group]]s and [[Mulliken]] notation for their irreducible representations) is used. </font>
| | {{Image|Refl parab.png|right|350px|Fig. 2. Reflection in a parabolic mirror}} |
| | Parabolic mirrors concentrate incoming vertical light beams in their focus. We show this. |
|
| |
|
| The '''Jahn-Teller effect''' is the distortion of a highly symmetric—but non-linear—molecule to lower symmetry and lower energy. The effect occurs if the molecule is in a degenerate state of definite energy, that is, if more than one wave function is [[eigenfunction]] of the [[molecular Hamiltonian]] with the same energy. In other words, energy degeneracy of a state implies that there are two or more orthogonal wave functions describing the state. Due to Jahn-Teller distortion, the molecule is lowered in symmetry and the energy degeneracy is lifted. Some of the wave functions obtain lower energy, while others obtain higher energy by the distortion.
| | Consider in figure 2 the arbitrary vertical light beam (blue, parallel to the ''y''-axis) that enters the parabola and hits it at point ''P'' = (''x''<sub>1</sub>, ''y''<sub>1</sub>). The parabola (red) has focus in point ''F''. The incoming beam is reflected at ''P'' obeying the well-known law: incidence angle is angle of reflection. The angles involved are with the line ''APT'' which is tangent to the parabola at point ''P''. It will be shown that the reflected beam passes through ''F''. |
|
| |
|
| The effect is named after [[Hermann Jahn|H. A. Jahn]] and [[Edward Teller|E. Teller]] who predicted it in 1937.<ref>H. A. Jahn and E. Teller, ''Stability of Polyatomic Molecules in Degenerate Electronic States'', Proc. Royal Soc. vol. '''161''', pp. 220–235 (1937)</ref> It took some time before the effect was experimentally observed, because it was masked by other molecular interactions. However, there are now numerous unambiguous observations that agree well with theoretical predictions. These range from the excited states of the simplest non-linear molecule H<sub>3</sub>, through moderate sized organic molecules, like ions of substituted [[benzene]], to complex [[crystal]]s and localized impurity centers in solids.
| | Clearly ∠''BPT'' = ∠''QPA'' (they are vertically opposite angles). Further ∠''APQ'' = ∠''FPA'' because the triangles ''FPA'' and ''QPA'' are congruent and hence ∠''FPA'' = ∠''BPT''. |
|
| |
|
| | | We prove the congruence of the triangles: By the definition of the parabola the line segments ''FP'' and ''QP'' are of equal length, because the length of the latter segment is the distance of ''P'' to the directrix and the length of ''FP'' is the distance of ''P'' to the focus. The point ''F'' has the coordinates (0,''f'') and the point ''Q'' has the coordinates (''x''<sub>1</sub>, −''f''). The line segment ''FQ'' has the equation |
| ==Explanation==
| | :<math> |
| The Jahn-Teller effect is best explained by an example. Consider the square homonuclear molecule in the middle of Fig. 1. Its symmetry group is D<sub>4h</sub>. Consider two electronic wave functions that together span the irreducible representation ''E''<sub>''u''</sub> of this group and assume that the molecule is in the corresponding electronic state. One wave function transforms as ''x'' and will be denoted by |''X''⟩. Its partner transforms as ''y'' and is denoted by |''Y''⟩. In the case of the perfect square both have the same energy <math>\varepsilon_{E}</math>.
| | \lambda\begin{pmatrix}0\\ f\end{pmatrix} + (1-\lambda)\begin{pmatrix}x_1\\ -f\end{pmatrix}, \quad 0\le\lambda\le 1. |
| | | </math> |
| In the middle of Fig.1 a [[normal mode]] ''Q'' (''v''<sub>4</sub>) is indicated by red arrows, explicitly it is
| | The midpoint ''A'' of ''FQ'' has coordinates (λ = ½): |
| | :<math> |
| | \frac{1}{2}\begin{pmatrix}0\\ f\end{pmatrix} + \frac{1}{2}\begin{pmatrix}x_1\\ -f\end{pmatrix} = |
| | \begin{pmatrix}\frac{1}{2} x_1\\ 0\end{pmatrix}. |
| | </math> |
| | Hence ''A'' lies on the ''x''-axis. |
| | The parabola has equation, |
| | :<math> |
| | y = \frac{1}{4f} x^2. |
| | </math> |
| | The equation of the tangent at ''P'' is |
| | :<math> |
| | y = y_1 + \frac{x_1}{2f} (x-x_1)\quad \hbox{with}\quad y_1 = \frac{x_1^2}{4f}. |
| | </math> |
| | This line intersects the ''x''-axis at ''y'' = 0, |
| :<math> | | :<math> |
| Q = -\Delta x_1 + \Delta x_3 + \Delta y_2 - \Delta y_4,
| | 0 = \frac{x_1^2}{4f} - \frac{x_1^2}{2f} + \frac{x_1}{2f} x |
| | \Longrightarrow \frac{x_1}{2f} x = \frac{x_1^2}{4f} \longrightarrow x = \tfrac{1}{2}x_1. |
| </math> | | </math> |
| where the deviations of the atoms are of the same length ''q''. For the record: ''Q'' transforms as ''B''<sub>''2g''</sub> of D<sub>4h</sub>. When ''q'' is positive, the molecule is elongated along the ''y''-axis; this is the leftmost molecule in Fig. 1. Similarly, negative ''q'' implies an elongation along the ''x''-axis (the rightmost molecule). The case ''q'' = 0 corresponds to the perfect square.
| | The intersection of the tangent with the ''x''-axis is the point ''A'' = (½''x''<sub>1</sub>, 0) that lies on the midpoint of ''FQ''. The corresponding sides of the triangles ''FPA'' and ''QPA'' are of equal length and hence the triangles are congruent. |
| | |
| The distorted molecules are of D<sub>2h</sub> symmetry; D<sub>2h</sub> is an [[Abelian group]] that has—as any Abelian group—only one-dimensional irreducible representations. Hence all electronic states of the distorted molecules are non-degenerate. The function |''X''⟩ transforms as B<sub>3u</sub> and |''Y''⟩ transforms as B<sub>2u</sub> of D<sub>2h</sub>. Let the respective energies be <math>\varepsilon_{X}</math> and <math>\varepsilon_{Y}</math>. Although group theory tells us that the energies are different, without explicit calculation it is not a priori clear which energy is higher. Let us assume that for positive ''q'' : <math>\varepsilon_{X} < \varepsilon_{Y}</math>. This is shown in the energy level scheme in the bottom part of Fig. 1. An important observation is that the leftmost and rightmost molecules in the figure are essentially the same, they follow from each other by rotation over ±90° around the ''z''-axis (a rotation of the molecules in the ''xy''-plane). The ''x'' and ''y'' direction are interchanged between the left- and rightmost molecules by this rotation. Hence the molecule distorted with negative ''q''-value has: <math>\varepsilon_{X} > \varepsilon_{Y}</math>.
| |
| | |
| For small ''q''-values it is fair to assume that both <math>\varepsilon_{X}</math> and <math>\varepsilon_{Y}</math> are quadratic functions of ''q''. They are shown in Fig. 2. For ''q'' = 0 the curves cross and <math>\varepsilon_{X} = \varepsilon_{Y} \equiv \varepsilon_{E}</math>. The crossing point, corresponding to the perfect square, is clearly not an absolute minimum; therefore, the totally square symmetric configuration of the molecule will not be a stable equilibrium for the degenerate electronic state. At equilibrium, the molecule will be distorted from a square, and its energy will be lowered.
| |
| | |
| The above arguments are not restricted to square molecules. With the exception of linear molecules, which show [[Renner-Teller effect]]s, all polyatomic molecules of sufficiently high symmetry to possess spatially degenerate electronic states will be subject to the Jahn-Teller instability. The proof, as given by Jahn and Teller, proceeds by application of point group symmetry
| |
| principles.
| |
| | |
| ==Reference==
| |
| <references />
| |
Parabolic mirror
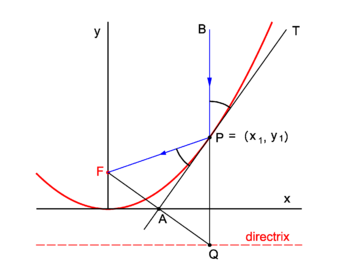
PD Image Fig. 2. Reflection in a parabolic mirror
Parabolic mirrors concentrate incoming vertical light beams in their focus. We show this.
Consider in figure 2 the arbitrary vertical light beam (blue, parallel to the y-axis) that enters the parabola and hits it at point P = (x1, y1). The parabola (red) has focus in point F. The incoming beam is reflected at P obeying the well-known law: incidence angle is angle of reflection. The angles involved are with the line APT which is tangent to the parabola at point P. It will be shown that the reflected beam passes through F.
Clearly ∠BPT = ∠QPA (they are vertically opposite angles). Further ∠APQ = ∠FPA because the triangles FPA and QPA are congruent and hence ∠FPA = ∠BPT.
We prove the congruence of the triangles: By the definition of the parabola the line segments FP and QP are of equal length, because the length of the latter segment is the distance of P to the directrix and the length of FP is the distance of P to the focus. The point F has the coordinates (0,f) and the point Q has the coordinates (x1, −f). The line segment FQ has the equation

The midpoint A of FQ has coordinates (λ = ½):

Hence A lies on the x-axis.
The parabola has equation,

The equation of the tangent at P is

This line intersects the x-axis at y = 0,

The intersection of the tangent with the x-axis is the point A = (½x1, 0) that lies on the midpoint of FQ. The corresponding sides of the triangles FPA and QPA are of equal length and hence the triangles are congruent.





