User talk:Paul Wormer/scratchbook1: Difference between revisions
imported>Paul Wormer |
imported>Paul Wormer |
||
| Line 8: | Line 8: | ||
{{Image|Jahn-Teller.png|right|400px|Fig. 1 <small> The middle molecule at the top is square planar (point group D<sub>4h</sub>). The red arrows indicate a stretch-type normal mode and the rhombus-shaped molecules on either side are obtained by deforming the middle molecule along the normal mode. The degenerate electronic energy <math>\scriptstyle \mathcal{E}_\mathrm{E}</math> is split by the deformation as shown at the bottom.</small>}} | {{Image|Jahn-Teller.png|right|400px|Fig. 1 <small> The middle molecule at the top is square planar (point group D<sub>4h</sub>). The red arrows indicate a stretch-type normal mode and the rhombus-shaped molecules on either side are obtained by deforming the middle molecule along the normal mode. The degenerate electronic energy <math>\scriptstyle \mathcal{E}_\mathrm{E}</math> is split by the deformation as shown at the bottom.</small>}} | ||
The Jahn-Teller effect is best explained by an example. Consider to that end the square homonuclear molecule in the middle of the top row of Fig. 1. The plane of the figure is the ''xy''-plane, with the ''y''-axis vertical and the ''x''-axis horizontal. The ''z''-axis points towards the reader. | The Jahn-Teller effect is best explained by an example. Consider to that end the square homonuclear molecule in the middle of the top row of Fig. 1. The plane of the figure is the ''xy''-plane, with the ''y''-axis vertical and the ''x''-axis horizontal. The ''z''-axis points towards the reader. | ||
The symmetry group of the middle molecule is D<sub>4h</sub>. Let there be two—in principle exact—electronic wave functions that together span the irreducible representation E<sub>''u''</sub> of this group | The symmetry group of the middle molecule is D<sub>4h</sub>. Let there be two—in principle exact—electronic wave functions that together span the irreducible representation E<sub>''u''</sub> of this group. One wave function transforms as an ''x''-coordinate under D<sub>4h</sub> and is denoted by |X ⟩. Its partner transforms as a ''y''-coordinate and is denoted by |Y ⟩. In the case of the perfect square both wave functions have the same energy denoted by <math>\mathcal{E}_\mathrm{E}</math>. | ||
To be more concrete, it may be helpful to mention that approximations for the wave functions can be obtained from two degenerate [[molecular orbital]]s |''x'' ⟩ and |''y'' ⟩ (together carrying E<sub>''u''</sub>) that are outside a closed-shell and share a single unpaired electron. A closed-shell wave function (a many-electron wave function consisting of doubly occupied molecular orbitals) is invariant under the group operations. The exact wave function |X ⟩ is then approximated by the closed-shell wave function times orbital |''x'' ⟩ containing the single electron, while |Y ⟩ is approximated by the closed-shell wave function times molecular orbital |''y''⟩ containing the electron. The orbital |''x'' ⟩ transforming as an ''x''-coordinate has the ''yz''-plane—a mirror plane—as a nodal plane, that is, the orbital vanishes in this plane and has plus sign to the right and minus sign to the left of the ''yz''-plane. Similarly the orbital |''y'' ⟩ has the ''xz''-plane as a nodal plane. | To be more concrete, it may be helpful to mention that approximations for the wave functions can be obtained from two degenerate [[molecular orbital]]s |''x'' ⟩ and |''y'' ⟩ (together carrying E<sub>''u''</sub>) that are outside a closed-shell and share a single unpaired electron. A closed-shell wave function (a many-electron wave function consisting of doubly occupied molecular orbitals) is invariant under the group operations. The exact wave function |X ⟩ is then approximated by the closed-shell wave function times orbital |''x'' ⟩ containing the single electron, while |Y ⟩ is approximated by the closed-shell wave function times molecular orbital |''y''⟩ containing the electron. The orbital |''x'' ⟩ transforming as an ''x''-coordinate has the ''yz''-plane—a mirror plane—as a nodal plane, that is, the orbital vanishes in this plane and has plus sign to the right and minus sign to the left of the ''yz''-plane. Similarly the orbital |''y'' ⟩ has the ''xz''-plane as a nodal plane. | ||
| Line 22: | Line 22: | ||
\mathcal{E}_\mathrm{X}(q) = \mathcal{E}_\mathrm{Y}(-q)\quad \hbox{and}\quad \mathcal{E}_\mathrm{X}(-q) = \mathcal{E}_\mathrm{Y}(q)\quad \hbox{with}\quad \mathcal{E}_\mathrm{X}(q) <\mathcal{E}_\mathrm{Y}(q)\quad\hbox{for}\quad q > 0. | \mathcal{E}_\mathrm{X}(q) = \mathcal{E}_\mathrm{Y}(-q)\quad \hbox{and}\quad \mathcal{E}_\mathrm{X}(-q) = \mathcal{E}_\mathrm{Y}(q)\quad \hbox{with}\quad \mathcal{E}_\mathrm{X}(q) <\mathcal{E}_\mathrm{Y}(q)\quad\hbox{for}\quad q > 0. | ||
</math> | </math> | ||
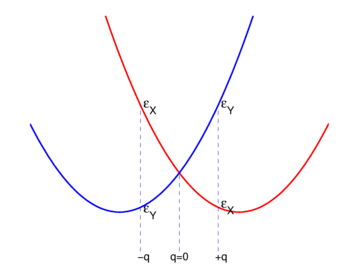
{{Image|J-T pot.png|left|350px|Fig. 2. | {{Image|J-T pot.png|left|350px|Fig. 2. Energies of state <nowiki>|X ⟩</nowiki> and state <nowiki>|Y ⟩</nowiki> as function of nuclear displacement ''q''. The case ''q'' <nowiki> = </nowiki>0 corresponds to the highly symmetric middle molecule of Fig. 1. }} | ||
For small ''q''-values it is reasonable to assume that both <math>\mathcal{E}_\mathrm{X}(q)</math> and <math>\mathcal{E}_\mathrm{Y}(q)</math> are quadratic functions of ''q''. The quadratic energy curves are shown in Fig. 2. For ''q'' = 0 the energy curves cross and <math>\mathcal{E}_\mathrm{X}(0) = \mathcal{E}_\mathrm{Y}(0) \equiv \mathcal{E}_\mathrm{E}</math>. The crossing point, corresponding to the perfect square, is clearly not an absolute minimum; therefore, the totally square symmetric configuration of the molecule will not be a stable equilibrium for the degenerate electronic state. At equilibrium, the molecule will be distorted along a normal mode away from square, and its energy will be lowered. This is the Jahn-Teller effect. | For small ''q''-values it is reasonable to assume that both <math>\mathcal{E}_\mathrm{X}(q)</math> and <math>\mathcal{E}_\mathrm{Y}(q)</math> are quadratic functions of ''q''. The quadratic energy curves are shown in Fig. 2. For ''q'' = 0 the energy curves cross and <math>\mathcal{E}_\mathrm{X}(0) = \mathcal{E}_\mathrm{Y}(0) \equiv \mathcal{E}_\mathrm{E}</math>. The crossing point, corresponding to the perfect square, is clearly not an absolute minimum; therefore, the totally square symmetric configuration of the molecule will not be a stable equilibrium for the degenerate electronic state. At equilibrium, the molecule will be distorted along a normal mode away from square, and its energy will be lowered. This is the Jahn-Teller effect. | ||
Revision as of 11:09, 21 February 2010
In molecular physics, the Jahn-Teller effect is the distortion of a highly symmetric—but non-linear—molecule to lower symmetry and lower energy. The effect occurs if the high-symmetric molecule would be in a degenerate state of definite energy, that is, if more than one wave function of the same energy would be eigenfunction of the molecular Hamiltonian. When energy degeneracy of a molecular state would arise—i.e., two or more orthogonal wave functions would describe the same molecular-energy eigenstate—it appears that a distorted, lower-symmetry, molecule will have lower, and accordingly more favorable, energy. Hence, by Jahn-Teller distortion, the molecule is lowered in symmetry and the energy degeneracy is lifted. Wave functions that would belong to the same energy in the high-symmetric molecule obtain different energies, some energies are lower, some higher.
The effect is named after H. A. Jahn and E. Teller who predicted it in 1937.[1] It took some time before the effect was experimentally observed, because it was masked by other molecular interactions. However, there are now numerous unambiguous observations that agree well with theoretical predictions. These range from the excited states of the simplest non-linear molecule H3, through moderate sized organic molecules, like cations of substituted benzene, to transition metal complexes and localized impurity centers in solids.
- The Jahn-Teller effect has a quantum mechanical origin and there is no classical-physics explanation of it. Therefore, some knowledge of quantum mechanics is prerequisite to the reading of this article. Further Schönflies notation for point groups and Mulliken notation for their irreducible representations is used.
Explanation
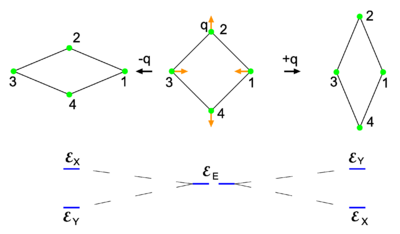
Fig. 1 The middle molecule at the top is square planar (point group D4h). The red arrows indicate a stretch-type normal mode and the rhombus-shaped molecules on either side are obtained by deforming the middle molecule along the normal mode. The degenerate electronic energy is split by the deformation as shown at the bottom.
The Jahn-Teller effect is best explained by an example. Consider to that end the square homonuclear molecule in the middle of the top row of Fig. 1. The plane of the figure is the xy-plane, with the y-axis vertical and the x-axis horizontal. The z-axis points towards the reader. The symmetry group of the middle molecule is D4h. Let there be two—in principle exact—electronic wave functions that together span the irreducible representation Eu of this group. One wave function transforms as an x-coordinate under D4h and is denoted by |X ⟩. Its partner transforms as a y-coordinate and is denoted by |Y ⟩. In the case of the perfect square both wave functions have the same energy denoted by .
To be more concrete, it may be helpful to mention that approximations for the wave functions can be obtained from two degenerate molecular orbitals |x ⟩ and |y ⟩ (together carrying Eu) that are outside a closed-shell and share a single unpaired electron. A closed-shell wave function (a many-electron wave function consisting of doubly occupied molecular orbitals) is invariant under the group operations. The exact wave function |X ⟩ is then approximated by the closed-shell wave function times orbital |x ⟩ containing the single electron, while |Y ⟩ is approximated by the closed-shell wave function times molecular orbital |y⟩ containing the electron. The orbital |x ⟩ transforming as an x-coordinate has the yz-plane—a mirror plane—as a nodal plane, that is, the orbital vanishes in this plane and has plus sign to the right and minus sign to the left of the yz-plane. Similarly the orbital |y ⟩ has the xz-plane as a nodal plane.
In the middle molecule shown in Fig. 1 an in-plane vibrational stretching normal mode Q of the planar molecule is indicated by red arrows. Explicitly, with respect to local, parallel, right-handed systems of axes, the red arrows represent the mode
where the deviations of the atoms are all of the same length |q|.[2] When q is positive, the molecule is elongated along the y-axis and compressed along the x-axis; this is the rightmost molecule in Fig. 1. Similarly, negative q implies a compression along the y-axis and an elongation along the x-axis (the leftmost molecule). The case q = 0 corresponds to the perfect square.
Both distorted rhombus-shaped molecules are of D2h symmetry; D2h is an Abelian group that has—as any Abelian group—only one-dimensional irreducible representations. Hence all electronic states of the distorted molecules are non-degenerate. The function |X ⟩ transforms as B3u and |Y ⟩ transforms as B2u of D2h. Let the respective energies be written as and . Group theory tells us that these energies are different, but without explicit calculation it is not a priori clear which of the two energies is higher. Let us assume that for positive q : . This is shown in the energy level scheme in the bottom part of Fig. 1. An important observation now is that the leftmost and rightmost distorted molecules are essentially the same, they follow from each other by an in-plane rotation over ±90° around the z-axis. The x and y direction are interchanged between the left- and rightmost molecules by this rotation. Hence the molecule distorted with negative q-value has the energies satisfying: . Summarizing,
For small q-values it is reasonable to assume that both and are quadratic functions of q. The quadratic energy curves are shown in Fig. 2. For q = 0 the energy curves cross and . The crossing point, corresponding to the perfect square, is clearly not an absolute minimum; therefore, the totally square symmetric configuration of the molecule will not be a stable equilibrium for the degenerate electronic state. At equilibrium, the molecule will be distorted along a normal mode away from square, and its energy will be lowered. This is the Jahn-Teller effect.
The above arguments are not restricted to square molecules. With the exception of linear molecules, which show Renner-Teller effects, all polyatomic molecules of sufficiently high symmetry to possess spatially degenerate electronic states will be subject to the Jahn-Teller instability. The proof, as given by Jahn and Teller, proceeds by application of point group symmetry principles; it rests on inspection of all molecular symmetry groups
Reference
- R. Englman, The Jahn-Teller Effect in Molecules and Crystals, Wiley, London (1972). ISBN-10: 0471241687 ISBN-13: 78-0471241683
- Isaac B. Bersuker, The Jahn-Teller Effect, Cambridge University Press (2006). ISBN-10: 0521822122 ISBN-13: 9780521822121