Magnetic moment: Difference between revisions
imported>John R. Brews (source) |
mNo edit summary |
||
| (19 intermediate revisions by one other user not shown) | |||
| Line 2: | Line 2: | ||
<br/> | <br/> | ||
{{TOC|right}} | {{TOC|right}} | ||
In physics, the '''magnetic moment''' of an object is a [[vector]] property, denoted here as '''m''', that determines the [[torque]], denoted here by '''τ''', it experiences in a [[magnetic flux density]] '''B''', namely '''τ''' = '''m × B''' (where '''×''' denotes the [[Vector product|vector cross product]]). As such, it also determines the change in [[potential energy]] of the object, denoted here by ''U'', when it is introduced to this flux, namely ''U'' = −'''m·B'''.<ref name=Bhatnagar | In physics, the '''magnetic moment''' of an object is a [[vector]] property, denoted here as '''m''', that determines the [[torque]], denoted here by '''τ''', it experiences in a [[magnetic flux density]] '''B''', namely '''τ''' = '''m × B''' (where '''×''' denotes the [[Vector product|vector cross product]]). As such, it also determines the change in [[potential energy]] of the object, denoted here by ''U'', when it is introduced to this flux, namely ''U'' = −'''m·B'''.<ref name=Bhatnagar/> | ||
==Origin== | ==Origin== | ||
| Line 12: | Line 8: | ||
===Macroscopic examples=== | ===Macroscopic examples=== | ||
{{Image|Motor.PNG|right|200px|Electric motor using a current loop in a [[magnetic induction|magnetic flux density]], labeled '''''B'''''}} | |||
The [[electric motor]] is based upon the torque experienced by a current loop in a magnetic field. The basic idea is that the current in the loop is made up of moving electrons, which are subect to the [[Lorentz force]] '''F''' in a magnetic field: | The [[electric motor]] is based upon the torque experienced by a current loop in a magnetic field. The basic idea is that the current in the loop is made up of moving electrons, which are subect to the [[Lorentz force]] '''F''' in a magnetic field: | ||
:<math>\mathbf F = -e \left( \mathbf {v \times B} \right) \ , </math> | :<math>\mathbf F = -e \left( \mathbf {v \times B} \right) \ , </math> | ||
where ''e'' is the [[Elementary charge|electron charge]] and '''v''' is the electron velocity. This force upon the electrons is communicated to the wire loop because the electrons cannot escape the wire, and so exert a force upon it. | where ''e'' is the [[Elementary charge|electron charge]] and '''v''' is the electron velocity. This force upon the electrons is communicated to the wire loop because the electrons cannot escape the wire, and so exert a force upon it. In the figure, the electrons at the left of the loop move oppositely to those at the right, so the force at the left is opposite in direction to that at the right. The magnetic field is in the plane of the loop, so the forces are normal to this plane, causing a [[torque]] upon the loop tending to turn the loop about an axis in the plane of the field.<ref name=motor/> According to the [[right-hand rule]], curling the fingers of the right hand about the loop in the direction of the forces twisting the loop makes the thumb point in the direction of the torque. In the figure the torque is therefore pointed in the plane of the ''B''-field and parallel to the two faces of the magnet. | ||
The torque exerted upon a current loop of radius ''a'' carrying a current ''I'', placed in a uniform magnetic flux density '''B''' at an angle to the unit normal '''û<sub>n</sub>''' to the loop is:<ref name=Pramanik/> | |||
The torque exerted upon a current loop of radius ''a'' carrying a current ''I'', placed in a uniform magnetic flux density '''B''' at an angle to the unit normal '''û<sub>n</sub>''' to the loop is:<ref name=Pramanik | |||
:<math>\boldsymbol \tau = \mathit I \mathbf {S \times B } \ , </math> | :<math>\boldsymbol \tau = \mathit I \mathbf {S \times B } \ , </math> | ||
| Line 38: | Line 27: | ||
The discussion splits naturally into two parts: [[kinematics]] and [[dynamics]]. | The discussion splits naturally into two parts: [[kinematics]] and [[dynamics]]. | ||
====Kinematics==== | ====Kinematics==== | ||
The kinematical discussion, which does not enter upon the physical origins of magnetism and its effects upon mechanics, deals with the classification of atomic states based upon symmetry. To emphasize this distinction, spin and orbital motion are considered here as distinct from spin angular momentum and orbital angular momentum. Although these ideas apply to nucleii and other particles, here attention is focused on electrons in atoms. | The kinematical discussion, which does not enter upon the physical origins of magnetism and its effects upon mechanics, deals with the classification of atomic states based upon symmetry. To emphasize this distinction, spin and orbital motion are considered here as distinct from spin angular momentum and orbital angular momentum. Although these ideas apply to nucleii and other particles, here attention is focused on electrons in atoms. | ||
=====Spherical symmetry===== | |||
</ | The symmetry analysis depends upon the environs of an atom. In situations where the spherical symmetry of an atom is little disturbed, spherical symmetry leads to the identification of spin '''S''' and orbital motion '''L''' and its combination '''J''' = '''L + S'''.<ref name=Weyl/> | ||
The electron has a spin. The resultant total spin '''S''' of an ensemble of electrons in an atom is the vector sum of the constituent spins '''s<sub>j</sub>''': | The electron has a spin. The resultant total spin '''S''' of an ensemble of electrons in an atom is the vector sum of the constituent spins '''s<sub>j</sub>''': | ||
| Line 55: | Line 45: | ||
:<math>\mathbf{J = L +S} \ .</math> | :<math>\mathbf{J = L +S} \ .</math> | ||
Assuming the atom remains symmetrical under rotations, '''J''', '''L''' and '''S''' are connected to this symmetry. The mathematical basis is the ''infinitesimal rotation'' from which finite rotations can be generated.<ref name=introduction/> For example, a rotation by angle α about the ''z''-axis is described by the matrix: | |||
:<math>\begin{pmatrix} | :<math>\begin{pmatrix} | ||
| Line 90: | Line 76: | ||
:<math>e^{i \alpha R_z} = 1 + i \alpha R_z + \frac{1}{2} \left( i \alpha R_z\right) ^2 + ... \ . </math> | :<math>e^{i \alpha R_z} = 1 + i \alpha R_z + \frac{1}{2} \left( i \alpha R_z\right) ^2 + ... \ . </math> | ||
If the three coordinate axes are labeled {''i, j, k ''} and the infinitesimal rotations about each of these axes are labeled {''R<sub>i</sub>, R<sub>j</sub>, R<sub>k</sub>''}, then these generators of infinitesimal rotations obey the ''commutation relations'':<ref name=Gottfried | If the three coordinate axes are labeled {''i, j, k ''} and the infinitesimal rotations about each of these axes are labeled {''R<sub>i</sub>, R<sub>j</sub>, R<sub>k</sub>''}, then these generators of infinitesimal rotations obey the ''commutation relations'':<ref name=Gottfried/> | ||
:<math> R_i R_j - R_jR_i = i \varepsilon_{ijk} R_k \ , </math> | :<math> R_i R_j - R_jR_i = i \varepsilon_{ijk} R_k \ , </math> | ||
for any choices of subscripts. Here ε<sub>ijk</sub> is the [[Levi-Civita symbol]] | for any choices of subscripts. Here ε<sub>ijk</sub> is the [[Levi-Civita symbol]]. | ||
These commutation relations now are viewed as applying in general, and while still considered as connected to rotations in three dimensional space, the question is opened as to what general mathematical objects might satisfy these rules. | |||
</ | A set of symbols with a defined sum and a product taken as a commutator of the symbols is called a [[Lie algebra]].<ref name=LieAlgebra/> In particular, one can construct sets of square matrices of various dimensions that satisfy these commutation rules; each set is a so-called ''representation'' of the rules. | ||
The matrices of dimension 2 are found from observation to be connected to the spin of the electron. One set of these matrices is based upon the [[Pauli spin matrices]]:<ref name= Reiher/> | |||
The matrices of dimension 2 are found from observation to be connected to the spin of the electron. One set of these matrices is based upon the [[Pauli spin matrices]]:<ref name= Reiher | |||
:<math>\sigma_x = \begin{pmatrix} | :<math>\sigma_x = \begin{pmatrix} | ||
| Line 136: | Line 103: | ||
with αβγ any combination of ''xyz''. | with αβγ any combination of ''xyz''. | ||
The | The matrix representation can be viewed as acting upon vectors in an abstract space. For example, a space with an odd number of dimensions (2ℓ+1) can be constructed from the [[spherical harmonics]] ''Y''<sub>ℓ</sub><sup>m</sup>, and their transformations under infinitesimal rotations.<ref name=Hladik/> | ||
The construction of irreducible matrices of any dimension at all is done as follows. If the generator of an infinitesimal rotation is labeled '''J''' where '''J''' = '''S''' or '''L''' or '''L + S''', for example, then the basis vectors in this space can be labeled by the integers ''j'' and ''m'' where ''m'' is restricted to the values { −''j'', −''j''+1, ... , ''j''−1, ''j'' }. Denoting a basis vector by |''j, m''⟩, one finds: | |||
:<math>J^2 |j, \ m \rangle = j(j+1) |j, \ m \rangle \ , </math> | |||
:<math>J_z|j, \ m \rangle = m |j, \ m \rangle \ . </math> | |||
Here ''J<sub>z</sub>'' generates an infinitesimal rotation about a direction chosen as the ''z''-axis, and ''J''<sup>2</sup> = ''J<sub>x</sub>''<sup>2</sup> + ''J<sub>y</sub>''<sup>2</sup> + ''J<sub>z</sub>''<sup>2</sup> is the so-called [[Casimir operator]].<ref name=Casimir/> In particular, these equations recover the Pauli matrices in two dimensions and the infinitesimal transformations of the ''Y''<sub>ℓ</sub><sup>m</sup> in (2ℓ+1) dimensions.<ref name=Blatt/> | |||
{{cite book |title= | Of course, the formalism has application to other elementary particles as well. | ||
=====Other symmetries===== | |||
The symmetry of a crystal is described by one of the [[space groups]], a set of transformations that includes certain particular rotations, reflections, translations, and operations called ''glides'' and ''screws''. Subgroups of the [[space groups]] are the so-called [[point groups]] that include only certain rotations and reflections, and apply to a subset of crystal symmetries. Thus, an atom in a crystal does not find itself in a situation of spherical symmetry. | |||
Nonetheless, the atom may maintain much of the behavior it exhibits under spherical symmetry, and that higher symmetry situation can be a good starting point for some materials. For others, the atomic symmetry is too distorted by the crystal environment, and a beginning point is based upon the [[electronic energy band]]s of the solid, which incorporate the space group symmetry.<ref name=Jones/> As described by Kubler:<ref name=Kubler1/> "We abandon the quantum numbers of the free atom states and try to work out a magnetic solution in the band picture." | |||
====Dynamics==== | |||
The dynamic aspect introduces the proportionality between magnetic moment and angular momentum using the [[gyromagnetic ratio]], and attempts to explain its origin based upon [[quantum electrodynamics]]. | |||
[[Angular momentum (quantum)|Angular momentum]] is introduced as proportional to the generator of an infinitesimal rotation, and is related to the same commutation relations, but with a proportionality factor of ℏ. Thus, in general ℏ'''J''' is an angular momentum, which clearly extends the idea of angular momentum far beyond the intuitive classical concept that applies in only three-dimensional space. | |||
The magnetic moment '''m'''<sub>S</sub> of a system of electrons with spin '''S''' is:<ref name=experiment/> | |||
:<math>\mathbf{m_S} = 2m_B \mathbf S \ , </math> | |||
and the magnetic moment '''m'''<sub>L</sub> of an electronic orbital motion '''L''' is: | |||
:<math>\mathbf{m_L} = m_B \mathbf{L} \ . </math> | |||
Here the factor ''m<sub>B</sub>'' refers to the [[Bohr magneton]], defined by: | |||
:<math>m_B = \frac{e \hbar}{2 m_e} \ , </math> | |||
with ''e'' = the [[Elementary charge|electron charge]], ℏ = [[Planck's constant]] divided by 2π, and ''m<sub>e</sub>'' = the electron mass. These relations are generalized using the ''g''-factor: | |||
:<math>\mathbf{m_J} = g m_B \ \mathbf J \ , </math> | |||
with ''g''=2 for spin ('''J''' = '''S''') and ''g''=1 for orbital motion ('''J''' = '''L''').<ref name=Poole/> | |||
As mentioned earlier, where both spin and orbital motion are present, they combine by vector addition:<ref name=Newton/> | |||
:<math>\mathbf{J = L +S} \ .</math> | |||
The magnetic moment of an atom of angular momentum ℏ'''J''' is | |||
:<math>\mathbf {m_J} = g m_B \mathbf J \ , </math> | |||
with ''g'' now the ''Landé ''g''-factor'' or spectroscopic splitting factor:<ref name=Singh/> | |||
:<math> g = \frac {3}{2} + \frac{S(S+1)-L(L+1)}{2J(J+1)} \ . </math> | |||
This form assumes the ''LS coupling scheme'' in which all the orbital angular momenta couple to form '''L''' and all the spins to form '''S'''. | |||
If an atom with this associated magnetic moment now is subjected to a magnetic flux, it will experience a torque due to the applied field. | |||
This approach is approximate because spin and orbital angular momentum are coupled (the so-called [[spin-orbit coupling]]), an important influence in heavier atoms or highly ionized atoms. For atoms where this coupling is strong, a scheme called ''jj-coupling'' is more accurate. In this scheme the orbital and spin momenta of each electron are combined separately and then all the individual '''j'''-values are added to get the total '''J'''.<ref name= Sathyanarayana/> | |||
Because atoms in solids experience a lower symmetry than isolated atoms, they may require a different treatment, for example, one based upon itinerant electrons.<ref name=Buschow/> | |||
==Notes== | |||
{{Reflist|refs= | |||
<ref name=Bhatnagar> | |||
{{cite book |title=A Complete Course in ISC Physics |author=V. P. Bhatnagar |url=http://books.google.com/books?id=2kh2LnCB6E4C&pg=PA246 |pages=p. 246 |isbn=8120902025 |year=1997 |publisher=Pitambar Publishing}} | |||
</ref> | |||
<ref name=Blatt> | |||
For example, see {{cite book |title=Theoretical nuclear physics |author=John M. Blatt, Victor F. Weisskopf |url=http://books.google.com/books?id=R3BzWYQqNGsC&pg=PA782 |pages=p. 782 |isbn=0486668274 |year=1991 |publisher=Courier Dover Publications |edition=Reprint of 1979 Springer-Verlag ed}} | |||
</ref> | |||
<ref name=Buschow> | |||
See, for example, {{cite book |title=Physics of magnetism and magnetic materials |chapter=Chapter 7: Itinerant-electron magnetism |author=K. H. J. Buschow, Frank R. Boer |url=http://books.google.com/books?id=e096qvuMhGsC&pg=PA63 |pages pp. 63 ''ff'' |isbn=0306474212 |year=2003 |publisher=Springer}} | |||
</ref> | |||
<ref name=Casimir> | |||
{{cite book |title=Groups and Symmetries: From Finite Groups to Lie Groups |author=Yvette Kosmann-Schwarzbach |chapter=§3.2: The Casimir operator |url=http://books.google.com/books?id=8Aa4uS6ZcJEC&pg=PA99 |pages=pp. 99 ''ff'' |isbn=0387788654 |year=2009 |publisher=Springer|edition=Stephanie Frank Singer translation}} | |||
</ref> | |||
<ref name=experiment> | |||
The measured magnetic moment of an electron differs slightly from the value ''g''=2 due to interaction with the quantum vacuum. See Newton, for example. | |||
</ref> | |||
<ref name=Gottfried> | |||
{{cite book |title=Quantum mechanics: fundamentals |author=Kurt Gottfried, Tung-mow Yan |url=http://books.google.com/books?id=8gFX-9YcvIYC&pg=PA77 |pages=p. 77 |isbn=0387955763 |publisher=Springer |edition=2nd ed |year=2003}} | |||
</ref> | </ref> | ||
<ref name=Hladik> | |||
{{cite book |title=Spinors in physics |author=Jean Hladik |url=http://books.google.com/books?id=25eTtXqLW8UC&pg=PA83 |pages=pp. 83''ff'' |chapter=§3.3.2 Spherical harmonics |isbn=0387986472 |year=1999 |publisher=Springer}} | {{cite book |title=Spinors in physics |author=Jean Hladik |url=http://books.google.com/books?id=25eTtXqLW8UC&pg=PA83 |pages=pp. 83''ff'' |chapter=§3.3.2 Spherical harmonics |isbn=0387986472 |year=1999 |publisher=Springer}} | ||
| Line 167: | Line 192: | ||
</ref> | </ref> | ||
<ref name=introduction> | |||
This development is close to that found in {{cite book |title=Quantum field theory demystified |author=David McMahon |url=http://books.google.com/books?id=SoAZVbdyY8AC&pg=PA58 |pages=pp. 58 ''ff'' |chapter=The special orthogonal group SO(N)|publisher=McGraw-Hill Professional |isbn=0071543821 |year=2008}} | |||
</ref> | |||
<ref name=Jones> | |||
The classic in the area of energy bands in solids is {{cite book |title=The theory of Brillouin zones and electronic states in crystals |author= H Jones|publisher=North Holland Publishing Company |year=1975 |editions=2nd ed|isbn=0720400279 |url=http://books.google.com/books?id=dH7XPwAACAAJ}}. A more current authoritative work is {{cite book |title=Electronic structure: Basic theory and practical methods ||author=Richard M Martin |publisher =Cambridge University Press |year=2004 |url=http://books.google.com/books?id=dmRTFLpSGNsC&printsec=frontcover|isbn=0521782856}} An exhaustive treatment of symmetry in solids is {{cite book |title=Representations of the crystallographic space groups: Irreducible representations, induced representations and corepresentations |author=Oleg Vladimirovich Kovalev |edition=Translation by HT Stokes and DM Hatch 2nd ed |url=http://books.google.com/books?id=Da7ET9jGcrMC&printsec=frontcover |isbn=2881249345 |year=1993 |publisher=CRC Press}} | |||
</ref> | </ref> | ||
<ref name=Kubler1> | |||
==== | {{cite book |title=Theory of Itinerant Electron Magnetism |author=Jurgen Kubler |pages=pp. 165 ''ff'' |isbn=0199559023 |publisher=Oxford University Press |year=2009 |url=http://books.google.com/books?id=ZbM0gHCcmaQC&pg=PA158 |edition=Revised ed}} | ||
</ref> | |||
<ref name=LieAlgebra> | |||
For a mathematical discussion see {{cite book |title=Group Theory: An Intuitive Approach |author=R. Mirman |chapter=§X.7 Angular momentum operators and their algebra |isbn=9810233655 |year=1997 |pages =pp. 292 ''ff'' |url=http://books.google.com/books?id=LFakv848R2oC&pg=PA292 |publisher=World Scientific Publishing Company}} Matrices satisfying the commutation rules are called a ''matrix representation'' of the Lie algebra. See {{cite book |title=Dynamical groups and spectrum generating algebras, vol. 1 |author=BG Adams, J Cizek, J Paldus |editor=Arno Böhm ''et al.'' |edition=Reprint of [http://books.google.com/books?hl=en&lr=&id=sGsR18jJujYC&oi=fnd&pg=PA1 article] in ''Advances in Quantum Chemistry'', vol. 19, Academic Press, 1987 |url=http://books.google.com/books?id=KANTJADFKG4C&pg=PA114 |pages=pp. 114 ''ff'' |chapter= §2.2 Matrix representation of a Lie algebra |isbn=9971501473 |year=1987 |publisher=World Scientific}} | |||
</ref> | </ref> | ||
{{cite book |title= | <ref name=motor> | ||
For a discussion of the operation of a motor based upon the Lorentz force, see for example, {{cite book |title=Drives and Control for Industrial Automation |author=Kok Kiong Tan, Andi Sudjana Putra |url=http://books.google.com/books?id=auGLxYZlvX4C&pg=PA48 |pages=pp. 48 ''ff'' |isbn=1848824246 |year=2010 |publisher=Springer}} | |||
</ref> | </ref> | ||
<ref name=Newton> | |||
{{cite book |title=Quantum physics: a text for graduate students |author=Roger G. Newton |url=http://books.google.com/books?id=uPAzSaCuAk4C&pg=PA162 |pages=p. 162 |isbn=0387954732 |publisher=Springer |year=2002}} | {{cite book |title=Quantum physics: a text for graduate students |author=Roger G. Newton |url=http://books.google.com/books?id=uPAzSaCuAk4C&pg=PA162 |pages=p. 162 |isbn=0387954732 |publisher=Springer |year=2002}} | ||
| Line 210: | Line 227: | ||
</ref> | </ref> | ||
<ref name=Poole> | |||
{{cite book |title=Electron spin resonance: a comprehensive treatise on experimental techniques |author=Charles P. Poole |url=http://books.google.com/books?id=P-4PIoi7Z7IC&pg=PA4 |isbn=0486694445 |year=1996 |publisher=Courier Dover Publications |edition=Reprint of Wiley 1982 2nd ed |pages=p. 4}} | |||
</ref> | |||
<ref name=Pramanik> | |||
{{cite book |title=Electromagnetism: Theory and applications |author=A. Pramanik |url=http://books.google.com/books?id=gnEEwy12S5cC&pg=PT240 |pages=pp. 240 ''ff'' |isbn=8120319575 |year=2004 |publisher=PHI Learning Pvt. Ltd.}} | |||
</ref> | |||
<ref name= Reiher> | |||
{{cite book |title=Relativistic quantum chemistry: the fundamental theory of molecular science |author=Markus Reiher, Alexander Wolf |url=http://books.google.com/books?id=u47v2YmR-P8C&pg=PA141 |pages=p. 141 |isbn=3527312927 |year=2009 |publisher= Wiley-VCH}} | |||
</ref> | </ref> | ||
<ref name= Sathyanarayana> | |||
{{cite book |title=Electronic absorption spectroscopy and related techniques |author=D. N. Sathyanarayana |chapter=§2.4 jj coupling |url=http://books.google.com/books?id=VLIUnG9YimMC&pg=PA40 |pages=pp. 40 ''ff'' |isbn=8173713715 |year= 2001 |publisher=Universities Press (India) Pvt. Ltd}} | |||
</ref> | |||
<ref name=Singh> | |||
{{cite book |title= | {{cite book |title=Introduction To Modern Physics |author=R. B. Singh |url=http://books.google.com/books?id=fd3jyXM-vfMC&pg=PA262 |pages=p. 262 |year=2008 |isbn=8122414087 |publisher=New Age International}} | ||
</ref> | </ref> | ||
<ref name=Weyl> | |||
The mathematics of this classification is explained masterfully in {{cite book |title=The theory of groups and quantum mechanics |author=Hermann Weyl |isbn=0486602699 |year=1950 |publisher=Courier Dover Publications |edition=Reprint of 1932 ed |url=http://books.google.com/books?id=jQbEcDDqGb8C&pg=PA185 |pages=pp. 185 ''ff'' |chapter=Chapter IV A §1 ''The representation induced in system space by the rotation group''}}. The application to atomic spectra is explained in great detail in the classic {{cite book |title=The theory of atomic spectra |author=EU Condon and GH Shortley |isbn=0521092094 |year=1935 |publisher=Cambridge University Press |chapter=Chapter III: ''Angular momentum'' |pages=pp. 45 ''ff'' |url=http://books.google.com/books?id=hPyD-Nc_YmgC&pg=PA45}} and its modern update {{cite book |title=Atomic spectra |author=Edward Uhler Condon, Halis Odabaşi |url=http://books.google.com/books?id=hzE8AAAAIAAJ&pg=PA136 |chapter=Chapter 3: Angular momentum |isbn=0521298938 |year=1980 |publisher=Cambridge University Press}}. | |||
</ref> | |||
}}[[Category:Suggestion Bot Tag]] | |||
Latest revision as of 16:01, 14 September 2024
In physics, the magnetic moment of an object is a vector property, denoted here as m, that determines the torque, denoted here by τ, it experiences in a magnetic flux density B, namely τ = m × B (where × denotes the vector cross product). As such, it also determines the change in potential energy of the object, denoted here by U, when it is introduced to this flux, namely U = −m·B.[1]
Origin
A magnetic moment may have a macroscopic origin in a bar magnet or a current loop, for example, or microscopic origin in the spin of an elementary particle like an electron, or in the angular momentum of an atom.
Macroscopic examples
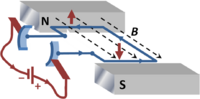
The electric motor is based upon the torque experienced by a current loop in a magnetic field. The basic idea is that the current in the loop is made up of moving electrons, which are subect to the Lorentz force F in a magnetic field:
where e is the electron charge and v is the electron velocity. This force upon the electrons is communicated to the wire loop because the electrons cannot escape the wire, and so exert a force upon it. In the figure, the electrons at the left of the loop move oppositely to those at the right, so the force at the left is opposite in direction to that at the right. The magnetic field is in the plane of the loop, so the forces are normal to this plane, causing a torque upon the loop tending to turn the loop about an axis in the plane of the field.[2] According to the right-hand rule, curling the fingers of the right hand about the loop in the direction of the forces twisting the loop makes the thumb point in the direction of the torque. In the figure the torque is therefore pointed in the plane of the B-field and parallel to the two faces of the magnet.
The torque exerted upon a current loop of radius a carrying a current I, placed in a uniform magnetic flux density B at an angle to the unit normal ûn to the loop is:[3]
where the vector S is:
Consequently the magnetic moment of this loop is:
Microscopic examples
Apart from macroscopic currents, at a fundamental level, magnetic moment is related to the angular momentum of particles: for example, electrons, nucleii, and so forth. In this discussion, focus is upon the electron and the atom.
The discussion splits naturally into two parts: kinematics and dynamics.
Kinematics
The kinematical discussion, which does not enter upon the physical origins of magnetism and its effects upon mechanics, deals with the classification of atomic states based upon symmetry. To emphasize this distinction, spin and orbital motion are considered here as distinct from spin angular momentum and orbital angular momentum. Although these ideas apply to nucleii and other particles, here attention is focused on electrons in atoms.
Spherical symmetry
The symmetry analysis depends upon the environs of an atom. In situations where the spherical symmetry of an atom is little disturbed, spherical symmetry leads to the identification of spin S and orbital motion L and its combination J = L + S.[4]
The electron has a spin. The resultant total spin S of an ensemble of electrons in an atom is the vector sum of the constituent spins sj:
Likewise, the orbital motions of an ensemble of electrons in an atom add as vectors.
Where both spin and orbital motion are present, they combine by vector addition:
Assuming the atom remains symmetrical under rotations, J, L and S are connected to this symmetry. The mathematical basis is the infinitesimal rotation from which finite rotations can be generated.[5] For example, a rotation by angle α about the z-axis is described by the matrix:
where the form following the arrow applies for very small angles α. The matrix Rz is called the generator of the z-rotation. The factor i is introduced so the finite rotation can be expressed in terms of this generator as a simple exponential:
as can be verified using the Taylor series:
If the three coordinate axes are labeled {i, j, k } and the infinitesimal rotations about each of these axes are labeled {Ri, Rj, Rk}, then these generators of infinitesimal rotations obey the commutation relations:[6]
for any choices of subscripts. Here εijk is the Levi-Civita symbol.
These commutation relations now are viewed as applying in general, and while still considered as connected to rotations in three dimensional space, the question is opened as to what general mathematical objects might satisfy these rules.
A set of symbols with a defined sum and a product taken as a commutator of the symbols is called a Lie algebra.[7] In particular, one can construct sets of square matrices of various dimensions that satisfy these commutation rules; each set is a so-called representation of the rules.
The matrices of dimension 2 are found from observation to be connected to the spin of the electron. One set of these matrices is based upon the Pauli spin matrices:[8]
which satisfy:
with αβγ any combination of xyz.
The matrix representation can be viewed as acting upon vectors in an abstract space. For example, a space with an odd number of dimensions (2ℓ+1) can be constructed from the spherical harmonics Yℓm, and their transformations under infinitesimal rotations.[9]
The construction of irreducible matrices of any dimension at all is done as follows. If the generator of an infinitesimal rotation is labeled J where J = S or L or L + S, for example, then the basis vectors in this space can be labeled by the integers j and m where m is restricted to the values { −j, −j+1, ... , j−1, j }. Denoting a basis vector by |j, m⟩, one finds:
Here Jz generates an infinitesimal rotation about a direction chosen as the z-axis, and J2 = Jx2 + Jy2 + Jz2 is the so-called Casimir operator.[10] In particular, these equations recover the Pauli matrices in two dimensions and the infinitesimal transformations of the Yℓm in (2ℓ+1) dimensions.[11]
Of course, the formalism has application to other elementary particles as well.
Other symmetries
The symmetry of a crystal is described by one of the space groups, a set of transformations that includes certain particular rotations, reflections, translations, and operations called glides and screws. Subgroups of the space groups are the so-called point groups that include only certain rotations and reflections, and apply to a subset of crystal symmetries. Thus, an atom in a crystal does not find itself in a situation of spherical symmetry.
Nonetheless, the atom may maintain much of the behavior it exhibits under spherical symmetry, and that higher symmetry situation can be a good starting point for some materials. For others, the atomic symmetry is too distorted by the crystal environment, and a beginning point is based upon the electronic energy bands of the solid, which incorporate the space group symmetry.[12] As described by Kubler:[13] "We abandon the quantum numbers of the free atom states and try to work out a magnetic solution in the band picture."
Dynamics
The dynamic aspect introduces the proportionality between magnetic moment and angular momentum using the gyromagnetic ratio, and attempts to explain its origin based upon quantum electrodynamics.
Angular momentum is introduced as proportional to the generator of an infinitesimal rotation, and is related to the same commutation relations, but with a proportionality factor of ℏ. Thus, in general ℏJ is an angular momentum, which clearly extends the idea of angular momentum far beyond the intuitive classical concept that applies in only three-dimensional space.
The magnetic moment mS of a system of electrons with spin S is:[14]
and the magnetic moment mL of an electronic orbital motion L is:
Here the factor mB refers to the Bohr magneton, defined by:
with e = the electron charge, ℏ = Planck's constant divided by 2π, and me = the electron mass. These relations are generalized using the g-factor:
with g=2 for spin (J = S) and g=1 for orbital motion (J = L).[15] As mentioned earlier, where both spin and orbital motion are present, they combine by vector addition:[16]
The magnetic moment of an atom of angular momentum ℏJ is
with g now the Landé g-factor or spectroscopic splitting factor:[17]
This form assumes the LS coupling scheme in which all the orbital angular momenta couple to form L and all the spins to form S.
If an atom with this associated magnetic moment now is subjected to a magnetic flux, it will experience a torque due to the applied field.
This approach is approximate because spin and orbital angular momentum are coupled (the so-called spin-orbit coupling), an important influence in heavier atoms or highly ionized atoms. For atoms where this coupling is strong, a scheme called jj-coupling is more accurate. In this scheme the orbital and spin momenta of each electron are combined separately and then all the individual j-values are added to get the total J.[18]
Because atoms in solids experience a lower symmetry than isolated atoms, they may require a different treatment, for example, one based upon itinerant electrons.[19]
Notes
- ↑ V. P. Bhatnagar (1997). A Complete Course in ISC Physics. Pitambar Publishing, p. 246. ISBN 8120902025.
- ↑ For a discussion of the operation of a motor based upon the Lorentz force, see for example, Kok Kiong Tan, Andi Sudjana Putra (2010). Drives and Control for Industrial Automation. Springer, pp. 48 ff. ISBN 1848824246.
- ↑ A. Pramanik (2004). Electromagnetism: Theory and applications. PHI Learning Pvt. Ltd., pp. 240 ff. ISBN 8120319575.
- ↑ The mathematics of this classification is explained masterfully in Hermann Weyl (1950). “Chapter IV A §1 The representation induced in system space by the rotation group”, The theory of groups and quantum mechanics, Reprint of 1932 ed. Courier Dover Publications, pp. 185 ff. ISBN 0486602699. . The application to atomic spectra is explained in great detail in the classic EU Condon and GH Shortley (1935). “Chapter III: Angular momentum”, The theory of atomic spectra. Cambridge University Press, pp. 45 ff. ISBN 0521092094. and its modern update Edward Uhler Condon, Halis Odabaşi (1980). “Chapter 3: Angular momentum”, Atomic spectra. Cambridge University Press. ISBN 0521298938. .
- ↑ This development is close to that found in David McMahon (2008). “The special orthogonal group SO(N)”, Quantum field theory demystified. McGraw-Hill Professional, pp. 58 ff. ISBN 0071543821.
- ↑ Kurt Gottfried, Tung-mow Yan (2003). Quantum mechanics: fundamentals, 2nd ed. Springer, p. 77. ISBN 0387955763.
- ↑ For a mathematical discussion see R. Mirman (1997). “§X.7 Angular momentum operators and their algebra”, Group Theory: An Intuitive Approach. World Scientific Publishing Company, pp. 292 ff. ISBN 9810233655. Matrices satisfying the commutation rules are called a matrix representation of the Lie algebra. See BG Adams, J Cizek, J Paldus (1987). “§2.2 Matrix representation of a Lie algebra”, Arno Böhm et al.: Dynamical groups and spectrum generating algebras, vol. 1, Reprint of article in Advances in Quantum Chemistry, vol. 19, Academic Press, 1987. World Scientific, pp. 114 ff. ISBN 9971501473.
- ↑ Markus Reiher, Alexander Wolf (2009). Relativistic quantum chemistry: the fundamental theory of molecular science. Wiley-VCH, p. 141. ISBN 3527312927.
- ↑ Jean Hladik (1999). “§3.3.2 Spherical harmonics”, Spinors in physics. Springer, pp. 83ff. ISBN 0387986472.
- ↑ Yvette Kosmann-Schwarzbach (2009). “§3.2: The Casimir operator”, Groups and Symmetries: From Finite Groups to Lie Groups, Stephanie Frank Singer translation. Springer, pp. 99 ff. ISBN 0387788654.
- ↑ For example, see John M. Blatt, Victor F. Weisskopf (1991). Theoretical nuclear physics, Reprint of 1979 Springer-Verlag ed. Courier Dover Publications, p. 782. ISBN 0486668274.
- ↑ The classic in the area of energy bands in solids is H Jones (1975). The theory of Brillouin zones and electronic states in crystals. North Holland Publishing Company. ISBN 0720400279. . A more current authoritative work is Richard M Martin (2004). Electronic structure: Basic theory and practical methods. Cambridge University Press. ISBN 0521782856. An exhaustive treatment of symmetry in solids is Oleg Vladimirovich Kovalev (1993). Representations of the crystallographic space groups: Irreducible representations, induced representations and corepresentations, Translation by HT Stokes and DM Hatch 2nd ed. CRC Press. ISBN 2881249345.
- ↑ Jurgen Kubler (2009). Theory of Itinerant Electron Magnetism, Revised ed. Oxford University Press, pp. 165 ff. ISBN 0199559023.
- ↑ The measured magnetic moment of an electron differs slightly from the value g=2 due to interaction with the quantum vacuum. See Newton, for example.
- ↑ Charles P. Poole (1996). Electron spin resonance: a comprehensive treatise on experimental techniques, Reprint of Wiley 1982 2nd ed. Courier Dover Publications, p. 4. ISBN 0486694445.
- ↑ Roger G. Newton (2002). Quantum physics: a text for graduate students. Springer, p. 162. ISBN 0387954732.
- ↑ R. B. Singh (2008). Introduction To Modern Physics. New Age International, p. 262. ISBN 8122414087.
- ↑ D. N. Sathyanarayana (2001). “§2.4 jj coupling”, Electronic absorption spectroscopy and related techniques. Universities Press (India) Pvt. Ltd, pp. 40 ff. ISBN 8173713715.
- ↑ See, for example, K. H. J. Buschow, Frank R. Boer (2003). “Chapter 7: Itinerant-electron magnetism”, Physics of magnetism and magnetic materials. Springer. ISBN 0306474212.