Plane (geometry): Difference between revisions
imported>Thomas Wright Sulcer (adding diagram) |
imported>Thomas Wright Sulcer (added picture) |
||
| Line 8: | Line 8: | ||
In [[Euclidean geometry]]: | In [[Euclidean geometry]]: | ||
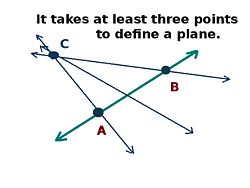
A '''plane''' is a [[surface (geometry)|surface]] on which a [[line (geometry)|line]] [[perpendicular (geometry)|perpendicular]] to a line which lies on that surface also falls entirely on the surface. It can be described by three [[point]]s which do not lie on the same line. A line is said to lie on a surface if its points fall on the surface. Surfaces can be [[parallel (geometry)|parallel]]. | A '''plane''' is a [[surface (geometry)|surface]] on which a [[line (geometry)|line]] [[perpendicular (geometry)|perpendicular]] to a line which lies on that surface also falls entirely on the surface. It can be described by three [[point]]s which do not lie on the same line. A line is said to lie on a surface if its points fall on the surface. Surfaces can be [[parallel (geometry)|parallel]]. | ||
[[Image:CrumpleEarth.jpg|thumb|250px|alt=Picture of a crumpled earth.|If this crumpled picture of the [[Earth]] was spread flat on a perfectly flat table, and the picture had absolutely no thickness, then it would be a plane.]] | |||
To distinguish between a plane and a surface think of a surface as a piece of paper (with no thickness) that could be twisted, or rolled, or crumpled up. A plane would be like that sheet of paper lying perfectly flat on a table. | To distinguish between a plane and a surface think of a surface as a piece of paper (with no thickness) that could be twisted, or rolled, or crumpled up. A plane would be like that sheet of paper lying perfectly flat on a table. | ||
Revision as of 17:17, 17 March 2010
Please create the "Talk page". Just click this Talk page link and save the page.
A plane is a surface such that any straight line linking any two points on the surface is part of the surface. A plane is made up of an infinite number of straight lines. At its simplest, a plane can be defined by a triangle whose vertices cannot be collinear.
In Euclidean geometry: A plane is a surface on which a line perpendicular to a line which lies on that surface also falls entirely on the surface. It can be described by three points which do not lie on the same line. A line is said to lie on a surface if its points fall on the surface. Surfaces can be parallel.

To distinguish between a plane and a surface think of a surface as a piece of paper (with no thickness) that could be twisted, or rolled, or crumpled up. A plane would be like that sheet of paper lying perfectly flat on a table.
A surface is usually designated by the names of the points which fall on it. Thus the surface has on it point A, point B, and point C is called surface ABC.
Plane figure
A plain figure is a combination of points and/or lines that fall on the same plane.
Rectilinear figure
A rectilinear figure is a plane figure consisting of straight lines only. Rectilinear figures include triangles and polygons.