Exponential function: Difference between revisions
imported>Dmitrii Kouznetsov m (→Generalization of exponential: misprint) |
imported>Dmitrii Kouznetsov (→Generalization of exponential: add figure. refs.) |
||
| Line 41: | Line 41: | ||
==Generalization of exponential== | ==Generalization of exponential== | ||
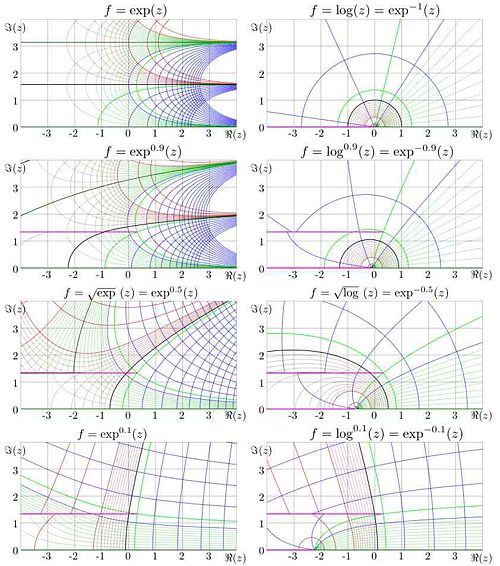
Notation <math>\exp_b</math> is used for the exponential with | [[Image:Sqrt(exp)(z).jpg|right|500px|thumb|<math>\exp^c(z)</math> in the complex <math>z</math> plane for some real values of <math>c</math>.]] | ||
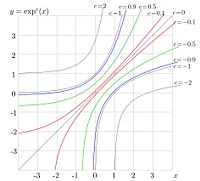
[[Image:Expc.jpg|right|200px|thumb|<math>\exp^c(x)</math> versus <math>x</math> for some real values of <math>c</math>.]] | |||
Notation <math>\exp_b</math> is used for the exponential with scaled argument; | |||
: <math>\exp_b(z)=b^z=\exp(\log(b) z)</math> | : <math>\exp_b(z)=b^z=\exp(\log(b) z)</math> | ||
| Line 59: | Line 62: | ||
:<math>F(z+1)=\exp_b(F(z))</math> | :<math>F(z+1)=\exp_b(F(z))</math> | ||
:<math>F(0)=1</math> | :<math>F(0)=1</math> | ||
:<math>F(z)~ \mathrm{ ~is~ holomorphic~ and~ bounded~ | :<math>F(z)~ \mathrm{ ~is~ holomorphic~ and~ bounded~ at}~ |\Re(z)|<1</math> | ||
The inverse function is defined with condition | The inverse function is defined with condition | ||
| Line 65: | Line 68: | ||
and, within some range of values of <math>z</math> | and, within some range of values of <math>z</math> | ||
: <math>F^{-1}\Big (F(z)\Big)=z</math> | : <math>F^{-1}\Big (F(z)\Big)=z</math> | ||
If in the notation <math>\exp_b^c</math> superscript is omitted, it is assumed to be unity; for example | |||
<math>\exp_b^1=\exp_b</math>. If the lower superscript is omitter, it is assumed to be <math>\mathrm{e}</math>, id est, | |||
<math>\exp^c=\exp_\mathrm{e}^c</math> | |||
==References== | |||
* Ahlfors, Lars V. (1953). Complex analysis. McGraw-Hill Book Company, Inc.. | |||
* H.Kneser. ``Reelle analytische L\"osungen der Gleichung <math>\varphi(\varphi(x))=\mathrm{e}^{x}</math> | |||
und verwandter Funktionalgleichungen''. Journal f\"ur die reine und angewandte Mathematik, <b> 187</b> (1950), 56-67. | |||
[[Category:Funcitons]] | |||
[[Category:Mathematica funcitons]] | |||
Revision as of 01:10, 29 October 2008
Exponential function or exp, can be defined as solution of differential equaiton
with additional condition
Exponential function is believed to be invented by Leonarf Euler some centuries ago. Since that time, it is widely used in technology and science; in particular, the exponential growth is described with such function.
Properties
exp is entire function.
For any comples and , the basic property holds:
The definition allows to calculate all the derrivatives at zero; so, the Tailor expansion has form
where means the set of complex numbers. The series converges for and complex . In particular, the series converge for any real value of the argument.
Inverse function
Inverse function of the exponential is logarithm; for any complex , the relation holds:
Exponential also can be considered as inverse of logarithm, while the imaginary part of the argument is smaller than :
While lofarithm has cut at the negative part of the real axis, exp can be considered
Number e
is widely used in applications; this notation is commonly accepted. Its approximate value is
- Failed to parse (syntax error): {\displaystyle {\rm e}=\exp(1) \approx 2.71828 18284 59045 23536}
Relation with sin and cos functions
Generalization of exponential
Notation is used for the exponential with scaled argument;
Notation is used for the iterated exponential:
For non-integer values of , the iterated exponential can be defined as
where is function satisfying conditions
The inverse function is defined with condition
and, within some range of values of
If in the notation superscript is omitted, it is assumed to be unity; for example . If the lower superscript is omitter, it is assumed to be , id est,
References
- Ahlfors, Lars V. (1953). Complex analysis. McGraw-Hill Book Company, Inc..
- H.Kneser. ``Reelle analytische L\"osungen der Gleichung
und verwandter Funktionalgleichungen. Journal f\"ur die reine und angewandte Mathematik, 187 (1950), 56-67.